Next: ``Ecology'' of Magnetic Rotators Up: Evolutionary Scenarios of Binary Previous: Semi-major axis change
Next: ``Ecology''
of Magnetic Rotators Up: Evolutionary
Scenarios of Binary Previous: Semi-major
axis change
Supernova explosion in a binary is treated as an instantaneous mass loss of the exploding star. We assume that an additional kick velocity is imparted to young neutron stars due to possible asymmetry of the collapse (see Section 5 for further discussion). In this case, the eccentricity and semi-major axis of the binary after the explosion can be straightforwardly calculated (Boersma, 1961[21]). We do this using the following scheme.
is calculated and kick vwlocity 
arbitrarily oriented in space is added to the orbital velocity,
and orbital angular momentum 
are computed; if the new total energy is negative, then the new semimajor
axis a' and eccentricity e'
are calculated by using the new
and
; if the total energy is positive (that is, the binary is unbound) spatial
velocities of each component are calculated. During the CE stage, an effective spiral-in of the
binary components occurs. This complicated process (first introduced by
Paczynsky, 1976[151]) is not fully understood
as yet, so we use the conventional energy consideration to find the binary
system parameters after the CE by introducing a parameter 
measuring what fraction of the system's orbital energy (between the beginning
and the end of the spiralling-in process) is transformed into the binding
energy (gravitational minus thermal) of the ejected envelope. Thus
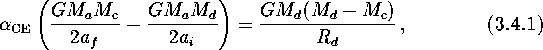
where 
is the mass of the core of the mass losing star of initial mass 
and radius 
(which is simply a function of the initial separation 
and the initial mass ratio 
), and no substantial mass growth is assumed for the accretor (see, however,
Chevalier, 1993[31]). The lower
is, the closer the binary becomes after the CE stage. We will show below
that the evolutionary scenario allows values of
over a wide range, 
-10.