Next: Extragalactic Gravitational Wave Background Previous: Transparency of the Stochastic
![]()
![]()
![]()
![]()
![]()
Next: Extragalactic
Gravitational Wave Background Previous: Transparency
of the Stochastic
Let the sky be observed with a detector having a pencil beam diagram
![]() across. The number of stars per frequency decade that is swept by the detector
is a function of celestial coordinates (say, galactic coordinates l,b):
across. The number of stars per frequency decade that is swept by the detector
is a function of celestial coordinates (say, galactic coordinates l,b):
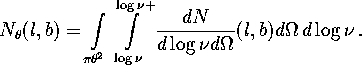
For a homogeneous distribution of stars over the sky this number is coordinate independent:
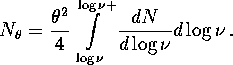
Here ![]() is defined as
is defined as
![]()
All sky ( ![]() steradian) corresponds to
steradian) corresponds to ![]() , and for small
, and for small ![]() this is just the halfwidth of the beam.
this is just the halfwidth of the beam.
The transparency of the background begins once ![]() ; this inequality provides us with the critical angular resolution
; this inequality provides us with the critical angular resolution ![]() the detector should have in order not to ``notice'' the background. To
obtain rough estimates we can use a ``homogeneous'' galaxy to find
the detector should have in order not to ``notice'' the background. To
obtain rough estimates we can use a ``homogeneous'' galaxy to find
Factor ![]() denotes
denotes
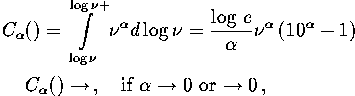
(below the dependence () will be omitted). Obviously, the red part is
of no interest (unless one observes a region of the sky very poor in stars),
and for the blue part one finds a critical frequency, ![]() , above which no stars occur in the region of the sky subtended by the
detector's beam. The function
, above which no stars occur in the region of the sky subtended by the
detector's beam. The function ![]() is shown in Figure 41.
is shown in Figure 41.
Figure 41: The regions of transparency of the GWB from different
objects in the angular resolution of the detector - critical frequency
![]() diagram. The GWB from a homogeneous model of our Galaxy (the total number
of stars is
diagram. The GWB from a homogeneous model of our Galaxy (the total number
of stars is ![]() ) is transparent below the line ``Our Galaxy''. The line marked ``sample
galaxy'' shows the transparency boundary for a Milky Way-type galaxy with
observed angular size
) is transparent below the line ``Our Galaxy''. The line marked ``sample
galaxy'' shows the transparency boundary for a Milky Way-type galaxy with
observed angular size ![]()
![]() . The break at 0.05 Hz is caused by the continuity limit of coalescing
binary WD at a rate of 1 per 100 year. The bottom curve shows the transparency
boundary for the GWB from
. The break at 0.05 Hz is caused by the continuity limit of coalescing
binary WD at a rate of 1 per 100 year. The bottom curve shows the transparency
boundary for the GWB from ![]() external galaxies. The upper horizontal line corresponds to the all-sky
detector (
external galaxies. The upper horizontal line corresponds to the all-sky
detector ( ![]() ). The hatched region corresponds to ``absolutely hopeless'' (Lipunov et
al., 1995a).
). The hatched region corresponds to ``absolutely hopeless'' (Lipunov et
al., 1995a).
It is clear from the figure that for an all-sky detector the Galaxy
becomes transparent above the frequency ![]() Hz. For a realistic LIGO detector network angular resolution of about
Hz. For a realistic LIGO detector network angular resolution of about
![]() this frequency reduces to
this frequency reduces to ![]() 2
2
![]()
![]() Hz.
Hz.
The isolines of the critical frequency on the (l,b)
sky map are presented in Figure 42
for the realistic galactic model described above and 10 percent of the
mass of the corona consisting of stars, seen by the ![]() -detector.
-detector.
Figure 42: Isolines of the critical frequency on the (l,b)-sky
map for the model of the Galaxy described in the text (Lipunov et al.,
1995a).